Metrology tools usually involve a tradeoff between slope acceptance and measurement speed. As a result, a compromise needs to be made between acceptable throughputs and adequate data coverage, when measuring machined parts with high slopes. In optical systems, for example, higher-NA objectives have higher slope acceptances, but they usually have narrow fields of view (FOV). This leads to individual measurements if the region of interest surpasses a single FOV.
Large-departure and recessed features pose an extra challenge - they impose a minimum working distance. This also involves a tradeoff with slope acceptance.
Coherence scanning interferometry (CSI) is used to measure machined parts. This method provides non-contact, areal topographical maps with typical single-measurement topography repeatability of less than one nanometer, on highly reflective and smooth surfaces. [1]-[2]. Usually, the selection of objective magnification ranges from about 1x to 100x. Preferably, the highest local slope θmax falls within the specular limit of the objective NA, so that the condition NA > sin (θmax) is satisfied. In actual practice, a lower-NA objective may be needed due to issues of cost and availability, or the limitations of field-size/throughput or minimum working distance.
Fortunately, it is possible to quantify slopes beyond the specular limit, if some light is scattered (usually by surface roughness) and the measurement is sufficiently sensitive. However, even in such conditions, it has often been difficult to measure high-slope parts by CSI.
Recent advancements in technology have considerably improved CSI’s baseline sensitivity and have enabled high dynamic-range operations. This allow measurement of high-slope or recessed features that were previously inaccessible, or larger FOVs for enhanced throughput.
Measurement and Analysis
Various machined parts were measured by means of a modern, commercial CSI microscope [3]. The operation of CSI is schematically shown in Figure 1. This involves scanning an interferometric object relative to the sample being measured, and creating localized interference patterns that define sample heights at all pixels of the camera. A single scan generates areal topographical data at corresponding positions of best focus over the full field of the camera. Any type of interferometric objective can be used, such as Mirau, Michelson, and wide-field or Linnik [4]-[5].
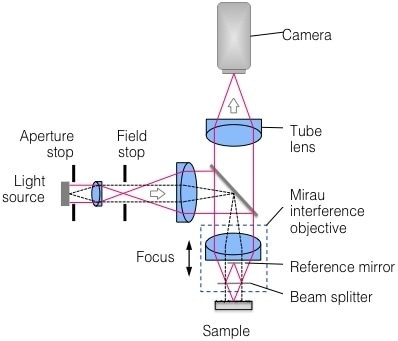
Figure 1. Schematic representation of Coherence Scanning Interferometry
The baseline data acquisition time for the results presented in this article is around 0.14 seconds per micron scanned. Raw height data is shown in all surface plots without any smoothing, masking or interpolation of missing data points. Particularly weak signals were detected using dynamic noise reduction (DNR), enabling a user-specified tradeoff between throughput and sensitivity while maintaining full vertical and vertical resolution [6].
Slopes Beyond the Specular Limit
Shown in Figure 2 is a diamond-turned cone with an included angle of 90°, where the cone has a relatively low surface roughness (Sa ~ 1.1 nm, as measured at normal incidence with a 10x Michelson objective), and an outermost diameter of 4 mm. In order to accommodate the 45° slopes within the specular limit, an objective with NA > 0.7 is required. In practice, this would involve stitching hundreds of FOV, with an undesirable throughput.
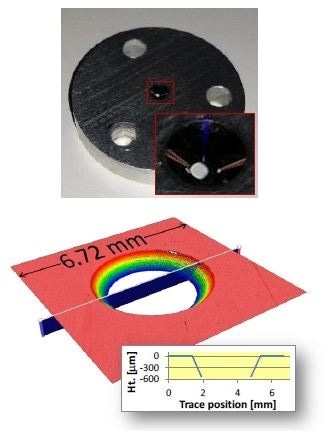
Figure 2. Measurement of 5-mm diameter diamond-turned 90° cone in a single FOV. Top: photograph of part; bottom: measured height map.
In order to measure the cone within a single FOV, a low-magnification objective with NA well below 0.7- is required. If a traditional CSI were used for such a measurement, only a small amount of data would be generated. However, we would learn that data at slopes above the specular limit allows scattered light to be detected easily.
Employing a 2.5x Michelson objective (NA = 0.075) along with 4x DNR (a 16x increase in baseline measurement time) results in almost-full data coverage. This data is perfect for measuring cone angle and roundness.
Applications, such as ball bearings and sealing surfaces, have spherical features with slopes of almost 90°. With increasing diameter, measurement of spherical features becomes complicated: high slopes are required for increasing working distance and FOV.
Shown in Figure 3 is a 3-mm-diameter fuel-injector sealing ball, as measured in a single FOV with a 5.5x Michelson (NA = 0.15). Almost-full data coverage is obtained for local slopes exceeding 60°. The same objective is used to measure surface roughness (Sa), which is approximately 0.1 µm.
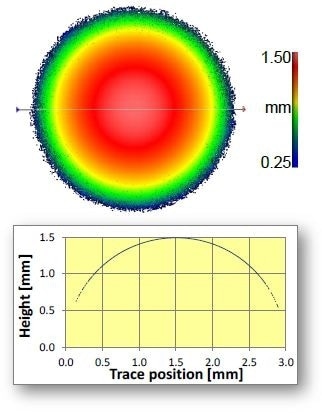
Figure 3. 3-mm-diameter sealing ball measured in a single FOV for local slopes up to 60°.
Access to larger FOVs is advantageous, even when stitching is required. The advantages include faster targeting of functional surfaces, registration to datum surfaces, and improved throughput. Form error is also decreased when less FOVs are used, and this error occurs due to stitching multiple slivers of data from smaller-aperture height maps.
Figure 4 shows a diamond end-mill [7] which is exposed to wear in three locations and generates local slopes of more than 70°. From this figure, it appears that stitching hundreds of high-NA measurements may be required. However, complete data coverage was obtained by stitching only ~ 20 FOVs employing a 20x Mirau objective (NA = 0.4, specular limit ~ 23°). The end result was a 0.4-µm lateral sampling (0.9-µm optical resolution) and nm-scale vertical resolution over the entire tool length, with a total measurement time of just 9 minutes.
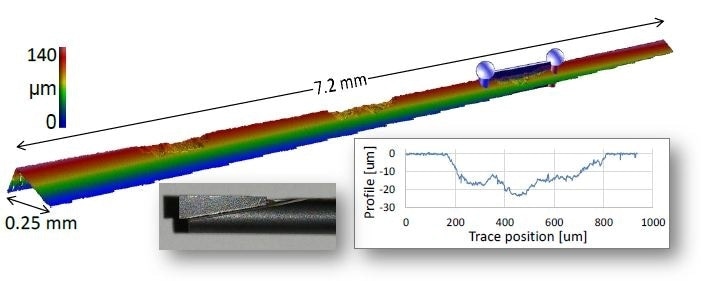
Figure 4. End-mill measured with 0.4 µm lateral sampling over 7.2-mm length and 145-µm scan range in about 9 minutes. Photograph of part is shown in lower left inset.
Effective measurement of slopes that exceed the specular limit depends on a number of factors, including the Sa of the sample and the spatial-frequency-dependent variations of the surface roughness.
Recessed High Slopes
High-slope features are occasionally recessed, at a distance below an adjacent feature. Common examples include shoulders along the exterior of a shaft and cones inside bores. Recessed features act as mounting or sealing surfaces, with corresponding crucial parameters such as radius, roundness, and angle.
In order to optically measure these critical parameters, an objective with sufficient working distance for accommodating the recess depth is required. This drastically reduces the options to lower-NA objectives, which forces measurements to be taken by using the available scattered light. Using a traditional CSI to measure extreme cases of recessed high slopes would be impractical.
Figure 5 displays a photograph of an unfinished fuel injector with surface roughness Sa ~ 1 µm. The highlighted region indicates a shoulder in which the fuel injector mounts against an engine block. The shoulder’s geometry is important to ensure proper sealing, and can be defined by the circumferential radius formed at the intersection of two steep-slope regions.

Figure 5. Photograph of fuel injector, with mounting shoulder region outlined in yellow
In the past, the measurement of the shoulder region would have required stitching many high-magnification measurements, apart from avoiding mechanical interference with the rest of the part. Figure 6 shows a measurement captured in a single FOV of a specialized 5x Michelson (NA = 0.12) with 40 mm working distance. Despite local slopes of up to 45°, almost-full data coverage is obtained.
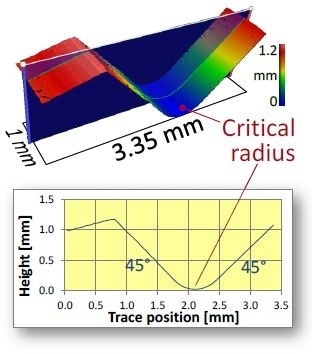
Figure 6. Measurement of shoulder region of fuel injector, highlighting radius critical for proper sealing with engine block.
Shown in Figure 7 is an electrical feed-through assembly that consists of a number of ~1-cm pins secured by a glass cladding that is recessed by ~1 mm inside a metal housing. Apart from the obstructing pins, the difficulties in the measurement of the glass profile include comparatively weak scattering due to moderate local slope of up to ~15°, and low roughness and refractive index.
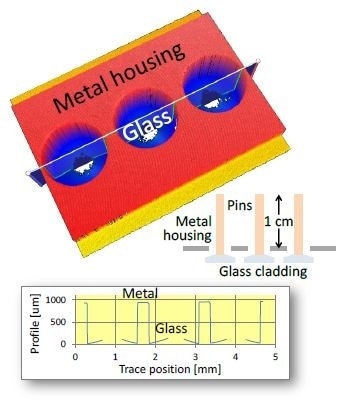
Figure 7. Electrical feed-through assembly. Of primary interest is the glass cladding, recessed below the metal housing along with pins extending ~1 cm beyond the housing. Blue regions in the height map correspond to glass, with missing data indicating the location of pins.
Almost-full data coverage of the glass cladding is obtained by employing a 0.15 NA 5.5x Michelson (NA = 0.15), with missing points corresponding mainly to the location of the covered pins. To efficiently manage the wide range of part reflectance (metal vs. tilted glass), a high dynamic range (HDR) mode was employed combining scans at varying light levels [6].
Near-Vertical Slopes
Maximum measurable slope is a function of objective NA together with the effective reflectivity of the surface being measured. So far, the examples presented in this article have been related to measurements using lower NA objectives. It would be interesting to see how high measured slopes can become at high values of NA.
The measured slope, for a hypodermic needle measured end-on in a single FOV using a 50x Mirau objective with NA = 0.55 and specular limit ~33°, is randomly close to the vertical (Figure 80. Data is obtained on all beveled surfaces, with existing slopes of 74° and 82° - data is also acquired along the near-vertical outer tube surface, where the slope is greater than 89°. As observed previously, there is neither any interpolation/masking nor any false data in the bore region.
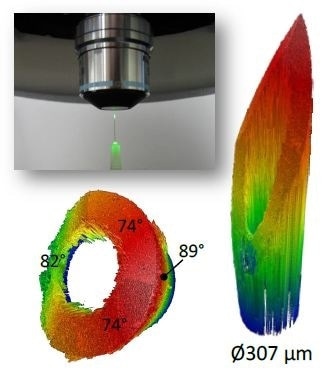
Figure 8. Hypodermic needle measured end-on in a single FOV. Upper left: photograph of setup. Right: obtained data over 1.8-mm scan range. Lower left: same data rotated to show measured slopes up to 89° and automatic identification of bore region.
Conclusion
High-slope and recessed features can now be measured through CSI, thanks to the recent technological progress to enhance sensitivity to weak interferometric signals. This was not possible before, using the technology. This technological advancement, in addition to improving baseline sensitivity, includes tools to further extend dynamic range, such as DNR and HDR [6]. Measurement of 89o slopes is now possible, which is evident from the examples.
On the whole, these enhancements enable wider latitude in objective selection, and allow operation at working distances for easy usage and larger FOVs, for enhanced throughput.
Acknowledgements
Key contributions and inputs from Eric Felkel, Nate Gilfroy, Mackenzie Massey and Dan Russano were beneficial to the article.
References
[1] de Groot P. Coherence Scanning Interferometry. In: Leach R, editor. Optical Measurement of Surface Topography. Berlin: Springer Verlag; 2011. p. 187- 208.
[2] ISO, [25178-604:2013(E): Geometrical product specification (GPS) – Surface texture: Areal – Nominal characteristics of non-contact (coherence scanning interferometric microscopy) instruments] International Organization for Standardization, Geneva (2013).
[3] Zygo Corporation, [NexView Optical Profiler], Specification sheet SS-0095 09/12 (2013).
[4] J. Biegen, X. Colonna de Lega and P. de Groot, "Wide-field interference microscopy for areal topography of precision engineered surface," Proc. ASPE annual meeting, paper 4111 (Boston, 2014).
[5] P. J. de Groot, L. L. Deck, J. F. Biegen and C. Koliopoulos "Equal-path interferometer", US Patent 8,045,175 (2011).
[6] Fay, M. F., Colonna de Lega, X., and de Groot, P. Measuring high-slope and super-smooth optics with high-dynamic-range coherence scanning interferometry. Proc. OSA, 2014: Paper 1981102.
[7] Thanks to Professor Chris Evans and Chris Tyler at the University of North Carolina at Charlotte (Mechanical Engineering and Engineering Science).

This information has been sourced, reviewed and adapted from materials provided by Zygo Corporation.
For more information on this source, please visit Zygo Corporation.